Heather Brooke Friends Name

creativity Beyond: Menelaos in Monterrey (1997)

National Contest Problem 2 (Monterrey 1997)

There are times when creativity is not enough (what is creativity? ), at least not creativity, in a populist version of the term, calling for the neglect of any theory for the sake of a sort of mystic illumination in the performance of intellectual tasks. I will argue for the proposition that expert knowledge cognizable maximizes efficiency in problem-solving tasks (or at least never is over) on the pretext of the next issue of the national competitive Mexican Mathematics Olympiad. Statement



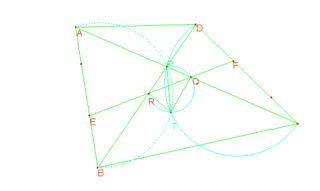
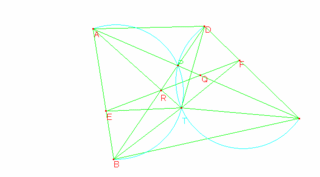
In a triangle ABC, P and P 'are two points on the side BC, CA Q & A on AB, so that AR / RB = BP / PC = CQ / QA = CP' / P'B. Let G be the centroid of the triangle ABC and K the intersection of AP 'with RQ. Show that P, G and K are collinear. Background Information

(a solution)

In the national contest of WMO (Mexican Mathematics Olympiad) in 1997 for the first time I knew that there was a theorem of Euclidean Geometry called Menelaus Theorem. As is known, the geometry in the school yard occupies a very marginal with a very marked bias in favor of algebra and analytical methods. And this seems to be the case throughout the world for some time now (perhaps since the advent of set theory in the early 1960).

In 1967, an American book that I got later to the intellectual shock of Monterrey (Coxeter and Greitzer, Geometry Revisited), the authors complained in the preface to the marginality of the geometry: "Perhaps the inferior status of geometry in the school curriculum comes from a lack of familiarity on the part of educators with the nature of the geometry and the advances that have taken place in its development. "

Anyway, Tamaulipas team of 6 teenagers with aspirations to enter the mathematical community came to Monterrey in the year of 97 with a background of knowledge completely out of context (which includes your coach, ie, me). An anonymous joke generated during the days after the contest read: "the Tamaulipas believe that all triangles are equilateral." (politically incorrect jokes are the most hurt, but possibly are the most commonly learn - neither good nor bad it's just that we were the Tamaulipas and the national competition that year, 1997.)

Valentina (17) was the one who told me, leaving examination of the first day and with a sad face and wonder: "2 out with Menelaus." But not because she had been resolved but because I heard in the corridors of mouth of fellow contestants that they had resolved itself. I lighten up something because at the meeting for discussion of issues that day so as to establish evaluation criteria, where delegates and advisers from all states first try to resolve the problems, none of the state advisory was even draw the figure describing the statement. Shame and disappointing surprise! And I thought that Val had received a good workout! Construction

in Figure guide

In diagrammatic reasoning (now I know) the diagram or image that guides the reasoning has to be generally free of irrelevant details and / or distracting the goal of reasoning. But in this case, to draw the figure describing the statement, the reasons given as data in the statement must have a particular k value, or the figure is impossible to draw.

Keep it Simple (KISS principle of design), warns the hacker slogan. So here I choose to keep the figure (e) Simple Stupides taking k = 1 / 2, ie the sides and Place trisecting points.



Digressions hints and clues about settlement

1) If verbalize the equal status of reasons it may be said that P, Q, R are based on the sides at the same rate, but also P 'part CB (BC reverse) in the same proportion as P part BC. This kind of symmetry is difficult to see at first glance (even to the trained eye). To the trained eye (in geometric problems Olympiad) likely this symmetry first acquires salience (something she suggests it is valuable scrutinize more closely) and only later is focused (looking implications derived
help the solution of the problem), and it if necessary to cover some details of the settlement plan.

However, after taking the picture, the cognized could be questioned whether the midpoint M of side BC is seen as the midpoint of PP 'only by the figure or is derivable from the condition. In my case, now that I address the problem years after that contest Monterrey, I explored this question at the end of the test as a need to come to a conclusion: that wanting to M was the midpoint of PP 'can prove to condition from the BP / PC = CP / P'B.

2) The application to demonstrate use Menelaus immediately suggests collinearity. But I suggest to anyone who knows the theorem and has experience with its instances of use. In 1997 I stayed just amazed that Valentina and I could do more partnerships that will link the data with geometric knowledge necessary for solving the problem (what to say to the solution ... I would have been happy if he had drawn the figure!) .

But suppose the cognized Menelaus can evoke from the statement. In that case, the following two tasks that immediately arise are a) finding the right triangle b) proceed to prove that the product of reason in the theorem of Menelaus is unity. (Note, incidentally, that the activation of the network of knowledge associated with Menelaus
- almost as if we had "zipped" in our mind - to cognizable provides an immediate action plan, your mind goes to work instead of keeping wandering in search of a useful idea - one that is wandering in the long sickening, especially when no useful idea comes.

3) Another clue to the solution that is included in the statement are the ratios RA / RB = CP '/ P'B (which almost immediately leads to the conclusion RP' parallel to AC) and QC / QA = CP ' / P'B (and seen to P'C is parallel to AB). These two ratios lead to the conclusion that the quadrilateral is parallelogram ARP'Q.

From here, cognizable eventually come to see that K is the intersection of diagonals which it could lead to another demonstration (No Menelaus) based on the idea that a medium must pass through the barycenter (centroid) - to which can be reached by a relatively random chain of associations, it also has to see that G is centroid of the triangle APP ' .

With random I mean that the action plan comes after the search for useful ideas (and there is a risk of failing to make them work even when they had sight) and not before as would be natural. With the evocation of the theorem of Menelaus, however, the plan is immediately and it will guide the action: it is like saying "What toolbox use for alignment problems?" - And the answer is and in the question itself ... if you had experience in instances of use of the theorem of Menelaus.

Solution (Menelaus)

Choosing the right triangle for the implementation of Menelaus is almost immediate: AMP is the triangle. " The next task is to show that (AG / GM) (MP / PP ') (P'K / KA) = 1. But this task is reduced to prove that MP / PP '= 1 / 2 for AG / GM = 2 / 1 for the attribute of the centroid location of the median and P'K / KA = 1 because the point K intersection of the diagonals of a parallelogram (the diagonals of a parallelogram bisect).

Here's where the need to demonstrate M is the midpoint of PP '(and not just BC). This can be proved by algebra. If k is the ratio BP / PC then from KPC and BP = CP '= kP'B can come to see that (k +1) PC = (k +1) P'B and therefore PC = P'B. Hence, PP '+ P'C = PP' + PB, ie P'C = PB. So BP + MP = MP '+ P'C and sees that PM = MP'.

Solution (without Menelaus)

Once knowing that M is the midpoint of PP 'show "atheoretical" (ie, elementary geometry) comes to mind from the image of the parallelogram ARPQ. Well, since G is also the centroid of the triangle APP '(the proportion MG / GA = 1 / 2 does not have to change), and since the diagonals of a parallelogram bisect each other, then the triangle median PK APP 'and must pass through G, ie, P, Q, K are collinear. Concluding Remarks



can be seen here (in the way the elementary solution arises or is suggested by the implementation of the solution requires more theory) that the solution "creative" is the least natural. This is because a plan to begin by saying "I will show that PK is average ..." is a plan unlikely. And also because the discovery that M is the midpoint of PP '(present in both shows) was a necessity in the plan Menelaus, and thus guided
by a goal, the ingredient that gives it natural.

Furthermore Menelaus cognizable maximizes efficiency by allowing complete the solution in less than an hour (I think). The moral I can draw from this problem is that the populism of many delegates and / or well-intentioned state coaches who argue that basic solutions Olympiad problems are always possible (as they are), hence to derive the conclusion (wrongly in my apparently) that adolescents who carry competitions require only natural creativity and basic skills, has the perverse effect of causing exactly what you want to avoid: the frustration of the boys.

In trying to avoid stress resulting from learning to demonstrate and use basic theorems and solve complex problems of competition only frustration Translates a week of competition, because there the children discover the futility of their tools in front of the complex tasks problems required a national contest for math Olympiad. [Although it could happen, as in my case the pageant of 97 coaches and delegates are innocent of populism, and simply be because, as Coxeter and Greitzer said, not familiar with the geometry (and other mathematical topics of competition).]

Adams County Ohio Hunting Leases

is said that projective geometry is a science born of art (Morris Kline, "The science born of art proved itself an art") and rightly so. The only problem is that the appreciation of art requires a long apprenticeship. The same is true of geometry. (Forget, even for a moment, the false promises of populism theoretical pedagogical fads in education - in its perennial quest for the philosopher's stone that would make all learning in a "blowout.")

The problem then be assumed known pose the following theorem:

If a triangle ABC are taken in BC points P, Q and R on AB CA so that the lines QR, RP and PQ intersect BC, CA, and AB in points P ', Q' and R 'respectively, then the points P', Q 'and R' are collinear if and only if the lines AP, BQ and CR are concurrent. (Exercise of it, prove it by applying the theorem of Menelaus.)

The problem I wish to raise here is:

If (under the conditions of the theorem above) the lines AP, BQ and CR are concurrent What can be said of lines AP, BQ 'and CR'?

Solution:

is easy to see that the answer is that they are concurrent. But this answer only comes after you get to see that besides the triangle PQR, there is another triangle that meets the PQR same conditions, except that is outside the triangle ABC. This triangle is the PQ'R '.

See

A -> P in BC .......................... A -> P

B-BC -> Q in CA .......................... B -> Q 'in CA

C -> A in AB .. ........................ C -> R 'in AB


AP, BQ and CR concur ......... ....... AP, BQ 'and CR' concur

......................... iff iff

'............................. AB.PQ AB.PQ = R '= R
BC.QR = P' ............................. BC.Q 'R' = P '= Q'.....
CA.RP ........................ CA.R 'P = Q

aligned ................ aligned ............

But R, Q and P 'are collinear by definition, since P' is intersection of BC and QR and therefore is on the line QR.

But what I want to emphasize here is that solving these problems (or prove these theorems) is a task that, plus it is very time consuming, requires knowledge of geometry have also needed to devote time for learning. And if the teen is believed the promise of populist educators will never be willing to spend more than 5 minutes to solve a math problem. From this point of view we can say that the school promotes ignorance.

Everybody Seems to Think I'm lazy
I do not mind, I think they're crazy Running everywhere at
Such a speed
Till They find, There's No Need

(I'm Only Sleeping, The Beatles)

Finally let me talk it I walked in Mexico City the week of the Congress of the Mexican Mathematical Society (24-28 October) and, after living three days in the abstract world in the Zocalo found a way to unwind and return to postmodernism: I chose the psychiatry of a sorcerer Aztec alternative.

What Can U Get Herpes From

desargueano IMO working Mérida 2005 (issue 5)

IMO, Mérida, solving problems as a sport

In July 2005 international mathematical community heard about Mexico (and the Mayan culture) through the city of Mérida hosted the International Mathematics Olympiad, maximum to which they aspire to reach teens who have decided to study mathematics from a sporting point of view.



Merida (and the Yucatan peninsula) evoke "natural" (at least here in Mexico) Maya culture, and in particular, Chichen-Itza (and the pyramid of Kukulkan) - a of impossible to ignore the traces of a glorious past, a vanished culture. (The photo is of José Muñoz Porras, who happens to accompany the delegation of Taiwan during the week of competition.)





But let me comment on the problem 5 of the competition, an issue that touched Jesus Rodriguez Viorato review and who promised to work that problem before he himself said in a talk by Congress of the Mexican Mathematical week from 24 to 28 October 2005.



The problem

Let ABCD be a convex quadrilateral with sides BC and AD equal and not parallel. E and F are points on sides BC and AD respectively such that BE = DF. The lines AC and BD intersect P, the lines DB and EF intersect at Q, the lines EF and AC intersect at R. Consider all triangles PRQ as E and F vary. Circuncírculos demonstrate that these triangles have another common point other than P.



Comments exploratory

The first thing you have to do in competition issues is an act of faith: what is being asked to prove is true. In this case, if requested to demonstrate that there is another common point to the PQR circuncírculos is that it exists. And the first task is to guess what that point is, tentatively characterize it then goes on to show that this is indeed the other point.

A good figure (well designed) can help. In fact, active exploration is needed on the initial figure. A good attitude for the solution of problems of competition is to reason as follows: if there is another point (and there must be since it is asking for your show) then that point must be one of those belonging to the configuration of the initial figure or likely to be characterized well in it.

For example, a point of intersection of two geometric objects can be generated from the figure. (It's like saying "! Looking for a mathematician!" And one that has to do is look at where the mathematicians tend to be - For example, at the Institute of Mathematics. I mean the places where we can find with probability one.)
EF
With two segments can locate the second point, call it T, circuncírculos for drawing the triangles PQR. But it remains to be seen how the objects is characterized by the figure (or other auxiliary constructed from those).



However, once located T graphically, one can somehow use the fact that (in a translation of the request) PRTQ configuration is cyclic quadrilateral (ie, if T The other point was then ... if we are to believe the veracity of the request). And that leads us to draw lines cyclic quadrilateral characteristics - such diagonals.

And what you want is to place T with respect to known geometric objects (in the settings given in the statement), not yet finished. You need more exploration. With a little luck (and perhaps experience similar problems - that I have and so I took much more time) can have the idea - a little more experimenting with the settings, perhaps because of the way it is T located in the figure - nothing to lose circuncírculos trace the APB and CPD, and it is there where does the discovery: T is the other point of intersection of these circuncírculos. (And the best part is that we can draw the whole theory of the geometry of the circle! - And in particular the cyclic quadrilateral)



targeting only certain key ideas of the show to avoid overloading this speech in text mode so geometric notation:

1) We need to find the points of interface between what we know (or can easily tell from the figure - with already Helper Objects -) and what we want knowledge, ie, show that PQTR is cyclical. An example: the angle is cool TPQ of TPA, but TPA is one of the angles of cyclic quadrilateral ABTP, the angle TPQ is thus a good interface.

2) It is easy to get lost with so many associations, and subsequent discoveries, angles (and their consequences), for example, in the figure are three isosceles triangles, but only two are used. So the compass should be the goal that you want to go, in this case the goal is to demonstrate PQRT cyclical (and shown from two angles to see that characteristic are equal). And if you have an angle (the QPT) then you must target the appropriate (the TRQ). Thus, the TRQ to the angle should be the compass.

3) The time immediately before reaching the angle TRQ is to discover that EBRT is cyclic quadrilateral, to which you get from the discovery that the isosceles BTD and ETF are similar. (This is done to see that the angles EBT - we already know from the beginning that is equal to the TPQ - and TRQ are supplementary angle of the ERT, and therefore are equal.

didactic digression (and design instruction in geometry)

From a teaching standpoint, the problem 5 of the IMO 2005 (and probably all the other 5, but those not yet explored) is already a repertoire of skills and knowledge in geometry that translate easily leave a training program.

And find one from the statement. Check out this: "Show that circuncírculos ..." The very term "circuncírculo" reflects primarily a teaching sequence: definition and understanding, from many years of construction, for example, the method of construction of a circuncírculo, its properties, the same for incírculos, excírculos, etc. And from there, the method of construction would lead to a dip straight from the triangle (medianas. bisectors, angle bisectors, etc..) And its associated theorems ...

Also in the statement, the phrase "as EF varies" (which should be understood "varies maintaining their properties") points to a training set reading skills - especially in reading "between the lines" of statements. And in recognition of the fact that, in a statement (and any speech), not everything is said, that certain holes should be filled by the reader.

On the discovery (the other point), guess what's the point T is a challenge - though perhaps not for many young people in Merida solved the problem in about two hours. The discovery phase aims at training forms and methods of exploration and experimentation with geometric configurations. Especially since a dynamic point of view (as EF varies). Particularly aimed at training in the use of geometry software (CBBRI, GSP, Cinderella, ...).

The way I discovered the other point may be illustrative. I used the CABRI (the French equivalent of Geometer's Sketch Pad). First drew the settings according to the statement and then circuncírculo for PQR. This did vary EF (as easy as moving the mouse one end). And I watched how he moved the circuncírculo.

But that did not help much to find the other point. So I drew on the settings you already had another line EF to have two circuncírculos and see what was the point remained fixed. Again moved with the mouse one end of a line EF and observed the dynamic behavior of the geometric configuration. So I saw that there was indeed another point (apart from P) that remained fixed during the movement of EF. But no more.

The check that there was another point he gave me some confidence (he had a psychological effect) but there was still characterize the fixed point T in terms of configuration objects. And perhaps it was the way T was located in the figure, with respect to other objects, which led me to more experimentation - all case, the geometry software, the experience costs almost nothing.

Another idea that helped me achieve and I guess before but I repeat it here: confidence in the axiom (axiom might be called geometric problem solving Olympiad) that T should be a starting point of the buildable and objects in the configuration.

It also has a psychological effect of discharge of anxiety, but also very real, and perhaps there could be a research project on this kind of statement, apparently mysterious but in which the clues are there in the words of those statements - the word circuncírculo I hovered in the head ...

So I built (with the CABRI) the PBL and CPD circuncírculos y. .. Oh revelation! Indeed, there was clearly that the point T is the other intersection of these circuncírculos.

In summary, I would like to stress here that the use of geometry software maximizes the efficiency in solving geometric problems. But above all the learning efficiency in solving geometric problems - since the contest only allowed the case of geometry.

Incidentally, and as a recognition astonished the young contestants, which is a feat of those boys - from those that solve the problem IMO 5 in 2005 - to have guessed the point T with ruler and compass (and have shown that this was indeed) in the short space of two or three hours - as they have 4 and half to solve the three problems. (Do not rule out the possibility that experienced similar problems, some have started the problem with "I'll show you the other intersection point is the other ..." and have saved the search and examination process to achieve conjecture.

Finally, let alone the end of these comments, the teaching or lesson that leaves the solution of a really complex problem, as is the problem 5 of the IMO 2005, is an experience life (or almost): the memory remains long in our minds. As I recall, that is, the configuration of relations between its objects, the demonstration plan, the intermediate steps ...

and clarified that what I present here is a reconstruction of a search that took me two days (and a third to draw up the reconstruction). And perhaps someone could say "what a waste of time!". And I would say "well ... everyone loses according to their own aesthetic preferences and trends ..." (What a pain! I'll have to catch up on the private lives of famous TELEVISA what antics have made REBEL those?! Gossip Pásenme please!)


Sincerely JMD in VL

What To Drink With Captain Morgan Tattoo

The difficult art of building pichoneras

The teenager fond of mathematics does not have to share the nostalgia of the teachers. So take this picture only as an invitation to visit my dokuwiki (Valentina did me the favor of staying in your virtual space). I have it a bit forgotten, but ... the cyber-readers visit it by clicking here



I followed these days by reading the book 500 challenges (see below) and I can recommend without reservation. The solution to the following problem (with comments inserted) is an example of the art of reasoning for clues (clues that only experience in problem solving taught to interpret). Previous comments



A logical principle of common sense, formalized as a technique for solving problems in Combinatorics and Discrete Mathematics, Box is called Pigeon Hole Principle or Principle (which can be translated as pigeonhole principle and the principle of pichoneras , respectively).

The principle says that if there are n pichoneras (nests) and n +1 pigeons (pigeons), then one of the nests will sleep 2 pigeons. (Formerly otherwise arise but for solution of problems with this approach is sufficient folk.) Let me

share an example from the Barbeau and Klamkin (E. Barbeau, M. Klamkin, W. Moser, Five Hundred Mathematical Challenges, MAA, 1995) to illustrate the fact that, although the principle is a truism, which is not obvious is how to build pichoneras do the trick of the show asked.

Problem. Any subset of size 7 drawn from {1,2 ,..., 126} can choose 2 elements b such that b / a is greater than 1 but not greater than 2. Are asked to prove this statement (proposition). Previous comments

2.

The first thing you should do the cognizable teenager who aspires to be a superstar in troubleshooting is experience exemplifying. And this in order to reach a full understanding of the statement. (Well, I think before that should be the mood and willpower - and fighting spirit - that immunity to the scarecrows - that is, cognizable should not panic.)

Tip:

Can you put the condition otherwise but equivalent - but more readable, ie, more understandable to you? Can you verbalize the equivalent condition? Problem

partner:

Trying to build a subset of size 7 with numbers 1 to 126 and that does not meet the given condition. (First it may be worth taking a subset of any size 7 and see if it meets the condition.)
short
Solution:

pichoneras The 6 {1.2}, {3,4,5,6}, {7 ,..., 14}, {15, .. ., 30}, {31 ,..., 62}, {63 ,..., 126} do the trick. (Note that each pichonera has the property that contains an x \u200b\u200bsuch that 2x is also on the pichonera. Moreover, they form the union of {1,2 ,..., 126}.)

Note 1: The

condition imposed on the problem can be put another way to multiply by the double inequality 1 < b/a < (o igual)2. Es decir, la condición sería equivalente a a < b < (o igual)2a. La cual ya es más fácil de verbalizar: "existen a y b tales que b está entre a y 2a (y, si acaso, b=2a)"

Note 2:

If we try to build a subset of size 7 to comply with the condition sooner or later we'll get a {1 , 3,7,15,31,63,127}. And this is illuminating and, as you can see that any element of the set has a double in the subset. From here you may eventually get the idea that
sets that meet the condition would be those in which there is an element that has its double in the set. (The pichoneras built into the solution meets this condition as adjusted - ie have only one element of that type.)

Note 3:

In problems of this kind - where the principle of the pichoneras trivially solved - how the wording suggests that pichoneras use. (Of course, to achieve capture the suggestion from the cognizable statement requires the expertise have resolved about 20 basic problems of this kind - through the experience acquired most of the codes.)

Note 4:

These problems can be frustrating due to the fact that you know the assertion is proved is true - for example, having built (in this case) the whole note 2, the revelation is accomplished ... "Of course! Not be otherwise, because would lack elements (127), but from there to prove it formally is a considerable distance.

Where Heather Harmon Go

How long does it take to cross Abbey Road? Finite Difference



Eleonora was in London in June and asked him to take a picture at Abbey Road (at the exact point they crossed the geniuses at Liverpool for the cover of that album and legendary) as a memorial to his father. Apart brought me a key chain. Thanks will be given.

But as this blog is math, let me pose this problem (adapted from the version of EJ Barbeau, MS Klamkin, and WOJ Moser, Five Hundred Mathematical Challenges, Mathematical Association of America, 1995). Eleonora

travels regularly to London and invariably takes the train back home, which arrives at the station of the town where you live at 5 PM. At the same time does his driver picks it up quickly and takes her home. Eleonora One day earlier took the train and arrives at the station at 4 PM. Instead of calling his driver, or wait until 5, Eleonora we walked toward home. On the road is your driver who picks it up quickly and takes her home, which come 20 minutes earlier than usual. A few weeks later, on another day, Eleonora takes an earlier train and arrives at the station at 4:30 PM. Like last time, we walked toward home. As before, the road is your driver who picks it up quickly and takes her home. How many minutes earlier than usual this time Eleonora comes home?

Solution: This is a basic problem extremely difficult. Possibly because there is a strong tendency to see it as a problem of speed: one seeks to calculate the speed of the car and Eleonora (the assumption is obviously constant speed - as in any problem of speed). The framing speed is desperate for "missing data": no distance or time.

The framing that does the trick of lighting is the distance. But gaps in terms of time. I mean, the house is at a distance of t minutes of the season. And although we do not know the value of t, we can see that the reference point is the path of the driver, who goes to the station and back in 2t minutes - usually. Leaves at 5-t returns home at 5 + t. (An image that can help visualize the situation is to imagine a long string of 2t. So if you are over 20 minutes on the rope ...)

After thinking for a while it is possible that enlightenment comes, the driver found Eleonora 10 minutes from the station. And as it usually reaches the station 5 then found her at 4:50 PM. It follows that what runs the car in 10 minutes, Eleonora in 50 runs. (The speed ratio is 1:5)

So when Eleanor arrived at the station at 4:30, the driver found her at a distance of 5 minutes (by car) from the station, ie at 4:55 PM, and got home 10 minutes earlier than usual.

(Note also that the station at 5 PM is the benchmark against which to estimate distances in time order.)


Sincerely JMD in VL

Italy Commercial Rabbit Cages

Join a community



Back in school again Maxwell (PEPE) plays the fool again. / Teacher gets annoyed.
Wishing to Avoid and Unpleasant / Sce, e, e, Jan, / She tells Max to stay When the class has gone away, / So I waits behind / Writing fifty times "I Must Not Be / So, or, or, or ...

MAXWELL'S SILVER HAMMER (The Beatles, Abbey Road)

Well, let me tell you that now I was reading the Hall & Knight, a legendary algebra book perhaps because it is too demanding on the reader. The issue: finite differences as a way to get the nth term of a sequence, and the sum of the series to n terms. And I wondered why such methods as are known in elementary school mathematics? I think the answer may be in the school mathematics culture: if nobody uses them, the predictable result is that public knowledge tends to die out, is the strength of the performance measures (standards) imposed by the community and their environment.

But let us put aside philosophy and social science (and the theory of evolution) and see how the method works.

If we have a succession U_1, u_2, u_3, ..., a pair differences of consecutive terms are called first-order differences to the differences of these differences are called second order, etc.. Thus, d_1 (U_1) = u_2-U_1, d_1 (u_2) u_2 = u_3-etc. And d_2 (U_1) = d_1 (u_2)-d_1 (U_1) d_2 (u_2) = d_1 (u_3)-d_1 (u_2), etc.

notation can be daunting, so it is better to see an example:

12 .... 40 .... 90 .... 168 .... 280 ... ....... 432
u_k
........................................ ... 28 ... 112 ..... 50 .... 78 ... 152 ................................. ............. d_1 (u_k)

...... 22 .... 28 ..... 34 ..... 40 ....... .......................................... d_2 (u_k)

......... 6 ...... 6 ...... 6 .......................... ........................... d_3 (u_k)

............. 0 ... ... 0 .............................................. .......... d_4 (u_k)


(I put points for alignment purposes.)

If you look at that u_2 = U_1 + d_1 (U_1) - and, in general, each term in the triangle is equal to its left over which is immediately below: 50 = 28 +22, for example - then achieved a formula for the nth term that follows the law of Newton's binomial coefficients in .

u_n = u_ (n-1) + d_1 (u_ (n-1)) and expands d_1 in terms of the law of formation described above. (Its expansion is left as an exercise.)

As an input (and support) we see the expansion and u_3 u_2:

u_2 = U_1 + d_1 (U_1)

u_3 = u_2 + d_1 (u_2) = [U_1 + d_1 ( U_1)] + [d_1 (U_1) + d_2 (U_1)] = U_1 +2 d_1 (U_1) + d_2 (U_1)

u_4 = u_3 + d_1 (u_3) = [U_1 +2 d_1 (U_1) + d_2 (U_1) ] + d_1 (u_2) + d_2 (u_2) U_1 ................=[

+2 d_1 (U_1) + d_2 (U_1)] +

.... ..................+ d_1 (U_1) + d_2 (U_1) +

................... ............+ d_2 (U_1) + d_3 (U_1)

Therefore: u_4 d_1 +3 = U_1 (U_1) +3 d_2 (U_1) + d_3 (U_1)

can see The coefficients are those of the binomial cube. If the expansion would continue to u_n = U_1 + C (n-1, 1) d_1 (U_1) + C (n-1, 2) d_2 (U_1) + C (n-1, 3) d_3 (U_1) + ...
(C (n-1, k) means "combinations of n-1 'k".) Is shown using mathematical induction. (Also left as an exercise.)

For the above example u_n = 12 +28 (n-1) +1 / 2 [22 (n-1) (n-2)] +1 / 6 [6 (n -1) (n-2) (n-3). That is, u_n = n ^ 3 +5 n ^ 2 +6 n. Sincerely JMD

in VL

note on mathematical notation: the unresolved problem of mathematical notation on the web requires me to use text mode; u_k means or subscript k, n ^ k means to the power n k; ...

Teac Ag-vs900 Receiver

On Thursday September 8, with the test protocol recepcional, I reached the end of the journey begun in January 2003 in Guadalajara. The Golden Fleece called Master of Communication I got after two years.

Can I Share Clothes If I Have Herpes

Monty Hall Paradox



make a deal (The Monty Hall Paradox)

Suppose that in a TV show you are participating and animator gives choice of three doors: what's behind the chosen is yours. Behind one of them is a new car, behind the other two a goat. You choose one of doors, say 1, and at that time (before opening) the driver, who knows what's behind each door, opens one of the other two, say 3, and shows a goat. And I asked "do you want to change your choice (opening the door 2)?" Do you should change?

This problem is paradoxical because common sense tells us that since we know nothing about what's behind the other two doors are the same chances of winning with which they choose with the other. But it can be shown that since the driver does know that behind the doors, it should change our decision. For a discussion of this point see http://math.ucsd.edu/ ~ crypto / Monty / montybg.html .

What implications does the driver know what's behind the doors? The most important is that the two two-thirds of the time (with probability 2 / 3) has no choice: the door that opens is the prize! Why, with probability 2 / 3? Well, eventually, for example of 100 players, about 67% of players choose a losing door (behind it is a goat) and in those cases the driver will have to show the contestant losing the second door (can not show the car). That is why the participants have more chances to win by changing your election to the third option as a strategy (ie, always, as a rule). Of course, if I had chosen the car door is going to lose, but that will happen with probability 1 / 3.

The Bottom Less Part In Harold And Kumar

This configuration of shapes and colors for the previous post. I decided to add it to the posts that try to be hoaxes. Moreover, it is a test to put pictures on the blog and try to make it more friendly (in the sense of friendly software). In the case of a post with a problem and see which configuration you get. Sincerely jmd

in vl

Can You Do Mma With Genital Warts

Guide Hatter happy (and yet, tragic) Troubleshooting

Note: it is understood that the guide is in the air (not that, but present) to solve mathematical problems in contemporary education - no one should be attributed (in particular swear the author was not me!)

Rule 1: Whenever possible, avoid reading the problem statement. Reading takes time and causes confusion. (Especially when it includes words not commonly used in youth culture - which is almost always ... ... what little kindness.)

Rule 2: Extract the numbers stated in the order they appear. Be careful with the numbers written in words.
Rule 3: If rule 2 gives you three or more numbers, the best chance is to add them all.
Rule 4: If two about the same size, then the rest is a good bet to achieve a good result.
Rule 5: If two numbers but of very different sizes, then divided, but if the division is more accurate then multiplies.
Rule 6: If you beat the problem requires using a formula then choose one with enough literals (letters) to use all the numbers given in the problem.
Rule 7: If rules 1 to 6 seem not work, make a desperate attempt: take the set of numbers found in regulation 2 random filling operations run about two pages. Note: do not forget to enclose a circle or box with five or 6 answers in each page ... chance and one of them is the answer, remember that you can get some points for trying, and are such attempts.

final note: the method is particularly effective when the teacher is among those who like to put "real" problems (the classic example - called "the age of the captain" -: on a boat there are 25 goats, 10 and two bastards kids, how old is the captain?). Under Rule 3, the answer is 37 - and a captain of 37 years is typical, as can be seen in old pirate movies. (I think it is best to avoid giving this answer explanations when answering "undecidable response given the irrelevance of the data.")

Best Bra At Victoria's Secret For Small Boobs

Review (Screener)

Problem:
Shows that 121 does not divide f (n) = n ^ 2 + 3n +5 for any natural number n.


Review:
Title: R_121_Criba In the petition "shows that 121 does not divide n ^ 2 + 3n + 5 for any natural n" the first thing that strikes our mind is the negative of the proposition (not divided) . How can I handle this? Ie how do you handle a negative proposition? If you ask "Prove that 121 divides a. .." like that somehow feels more manageable problem. But as it is posed the problem seems to us not computable, not processed by our cognitive systems. But it is. The force method Gross is daunting test for 1, 2, 3, ... A never ending story. "Induction? But if it is a denial? And our mind begins to spin in a whirlwind. But cool! If proposed in a contest should be resolvable. Moreover, it should be easy. First you have to look for clues of where you can enter. For any problem of competition must have a point of attack, a point where we can start thinking about your solution. The first clue lies in understanding what is asked. To avoid negative reading Let's think like I ask you to show that something is impossible (the 121 divide f (n)). If you know how to handle cases congruences reduce to 120 and you can apply brute force. And if well-organized calculations can verify the 120 cases in half an hour. But there's another track: 121 = 11 ^ 2. Why is it a clue? Well, because 11 is prime, and to verify that it is impossible that 121 divides f (n) is enough to prove that 11 does not divide twice to no n. The proposition calls for show can be translated as follows: two times 11 does not divide f (n). And here is now the key to the show: to divide into cases. (The 11 does not divide f (n), and if once divided not divide a second time.) Is the happy idea. The screening method. Another way to translate the problem and leads to the screen is to show that C = {n / 121 divides f (n)} is an empty set. The idea of \u200b\u200bscreening is to reduce on the search space. In principle, all cases must be analyzed. But with the screen first searched the n for which 11 divides f (n). This reduces the search space. Then in the set B = {n / 11 divided af}, we verify if 121 divides f for some element of B. First screen (method to determine if 11 divides f (n) for some n) as f (n) = n ^ 2 + 3n + 5 is not factorizable, it is factored leaving a multiple of 11 independent term. This requires adding and subtracting a constant c such that c + 5 is a multiple of 11 but at the same time, certain factors-c, say, c = ab, would have added the coefficient n in the equation, a + b = 3. (These formulas Vieta, a factorization method is applied intuitively and by trial and error: with practice the method considerably easier.) For this case we have (after several attempts): n ^ 2 + 3n +5 +28 - 28. The transformation sought, since n ^ 2 +3 n - 28 +33 meets the requirements and results in the factorization f (n) = (n + 7) (n - 4) + 33. And you can see that f is a multiple of 11 if and only if n = 4 + multiple of 11 (say n = 4 + 11k, with k an integer). Thus B = {n / n = 4 + 11k, k integer}. Second sieve The second sieve acts only on elements of B. For this is substituted into f (n) the generic element of B: f (4 + 11k) = (4 + 11k +7) (4 +11 k - 4) + 33 = 11 (k +1) +33 = 121k 11k (k + 1) +33.} And you can see that 121 will never divide f. Demonstrate general problem that does not divide p ^ 2 f (n) = n ^ 2 + (a + b) n + d for any natural n (p a prime). First we seek a constant c such that c + d = kp and at the same time-c = ab. The function would be f (n) = n ^ 2 + (a + b) n + ab + kp = (n + a) (n + b) + kp. Hence p divides f if and only if n = - a + rp or when n = - b + sp, with r and s integers. (Yes - a + b = p the problem is simplified and therefore only have to prove for-a + rp.) In the second screen would have: (-a + rp + a) (-a + rp + b) + kp = rp (r + 1) p + kp = r (r + 1) p ^ 2 + kp. Generating a problem Let p = 7, a = 2, b = -5, d = 4. Then the problem would be: to demonstrate that 49 does not divide n ^ 2 - 3n +4. Adding and subtracting 10 gives: n ^ 2 - 3n + 4 + 10 - 10 = n ^ 2 - 3n - 10 + 14 = (n + 2) (n - 5) + 14. So 7 divides f (n) if and only if n = -2 +7 k. But these numbers have f (-2 + 7k) = 49k (k + 1) + 14. And you can see that 49 does not divide f (n) for any n. Tip

Review

Best Shaving Cream 2010

Cut Solution (Screener)

Problem: shows that 121 does not divide f (n) = n ^ 2 + 3n +5 for any natural number n. Background:

In this problem you need to activate more of your proficiencies already acquired. There are several key concepts that should start operating. In order of importance: Essential

(to understand the statement):

1) divisibility 2) denial of a proposition

Needed (to try the demo) 3) demonstrations (logical argument)

Desirable:

4) congruences (for effectively manage issues of divisibility)

5) Vietta formulas (the "theoretical" remarkable products - for efficient factoring)

Tip Strategies

How Can I Make My Dune Buggy Go Faster

Strategies (Screener)

(Note: the solution does not help much if you do not know what the problem is. OK?)

Problem: Demonstrate that 121 does not divide f (n) = n ^ 2 + 3n + 5

Solution:
f (n) = n ^ 2 + 3n +5 +28 - 28 = (n + 7) (n - 4) +33

is clear then that 11 divides f (n) if and only if n is of the form 4 + 11k. But in that case, f (4 + 11k) = 121k (k + 1) + 33. Consequently 121

not divide f (n) for any n. Tip

Review