The northeastern Mexico VI Mathematics Olympiad (NMO) in Victoria
- by José Muñoz Delgado -
Event and Context
In an unprecedented symbolic act, the opening (Thursday, 21 September) ONM VI was the backdrop to announce the interest of the Ministry of Education of Tamaulipas (SET) and the Universidad Autonoma de Tamaulipas (UAT) in institutional strengthening school mathematics and its teaching in the state through the inter-agency project called "Learning Mathematics for Life."
The ONM is an event held every year as a contest prior to (and preparedness) the national competition for the Mexican Mathematics Olympiad (OMM). ONJ involved in Coahuila, Nuevo Leon and Tamaulipas, each with 15 teenagers shortlisted, and training for possible participation in the national contest of WMO.
The three delegations stayed at the Hotel Holiday Inn Express, the opening ceremony was held at the Centre of Excellence for the UAT, and the competition itself was on the premises of the UAM of Science, Education and Humanities (UAMCEH) for the TSU.
The Mathematics and Its Context Cares
For some reason (possibly sentimental) human beings are attracted to certain poles of attraction and communities formed around them (and, arguably, tribes). And following that human law (human too) a significant number
school mathematics teachers in Tamaulipas have gathered around the Math Olympiad devoting their time and effort to preparing teenagers for the competitions, no more pay than the mere satisfaction
see them rise in their level of knowledge and skills involved in math.
very particular in my case, I have dedicated much of my free time to the mathematics of competition since it started in Mexico, the organization of the Olympics Math (this year is 20, so take out the calculator ...) with some periods
forays into other fields (such as two and half years - 2003 to 2005 - I spent to get a master's degree in communication University of Guadalajara more expensive, thanks to PROMEP). But this year 2006 - and since late 2005 - I sold my watchtower math "with the slogan" The UAT should serve the state mathematically gifted adolescents. " Y. .. well ... against all odds ... a server and fellow teachers who joined my effort we convinced
UAT UAT and convinced the SET and the result is the interagency project launched at the opening of ONJ.
I think we're on the plastic moment in which the community of mathematicians Tamaulipas, related and supporters should join the project to flourish.
problems
contest the contest day I was walking in an effort to apply (to the Dra.Radmila Bulajich, president of the WMO Organizing Committee, who kindly accompanied us during the days of the event) transfer DEGETI Delegation to the UAT and therefore had not planned to be on the jury. But to get that day's contest UAMCEH facilities where the examination took place, the current company convinced me to be present at the jury, at least as an observer. Troubleshoot
decide the evaluation criteria for each test review, discuss the scores of cases unclear and / or controversial, casting hub scores, allocation of places (the medals) to competitors according to their performance, and report preparation. These are the tasks I was involved with the other jurors during the Friday, September 22 - from dawn to dusk and a little more.
For that the reader can form an idea of \u200b\u200bthe level of difficulty of the problems and the type of problems that the contestants have to solve, then the transcribed (the wording is not mine) and comment. Also transcribe the official solution, and drafted an alternative solution in the style of reasoning can of adolescents to address these difficult problems.
Problem 1. Show that there are infinite "m" such that n ^ 4 + m is a composite number for all n positive integer.
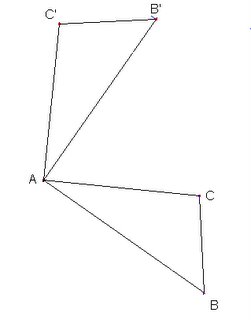
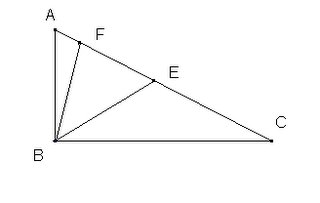
Problem 2. C1 and c2 are two circles that intersect at two points A and B. The tangent at A intersects c2 for c1 in C and the tangent to c1 and c2 A intersects D. A ray passing through A, inside the angle CAD, in M \u200b\u200bintersects c1 to c2 in N and the triangle ACD circuncírculo P. Prove that AM = NP.
Problem 3. It has a circumference perimeter 210. We have marked in the same 20 points, P1, P2, ..., P20, following the direction of clockwise so that the arc joining the point P1 to P2 has length 1, the arc connecting the point P2 P3 has the length 2, ... and so on, the arc joining the point P20 to P1 has length 20. Find all pairs of points scored such that the segment that joins them is the diameter of the circle.
General Comments on the Problems
The easy problem is 3. And this in the sense of being more visual and approachable by brute force - enough to know the meaning of "diameter." However, I should say that the problem 1 is easier that 3, but in another sense: the contestant must be convinced that intimidating statements on issues of competition tend to be easily "broken" translating into a language less esoteric.
In Problem 1, this translation could be "express m in terms of an integer r, such that n ^ 4 + f (r) is factorizable for any positive integer r." This translation has been to seek to complete the perfect square trinomial, difference of squares require and obtain the m requested. - The method could be called "backward inference" and illustrated in the alternative solution of this problem shown below.
Problem 1, however, Easy IMO the 1969 and would show that the judges considered it IMO international level. (See www.kalva.demon.co.uk/imo/ )
The really difficult problem is 2, a circle geometry problem. It is difficult in two respects. First you have to do (or generate - see alternative below) the binding idea and use it to build the solution, which requires familiarity with instances of use of the geometry of the circle - in particular, angles enrolled and semi-attached - to establish the similarities between pairs of triangles in the configuration. And this is assuming that the competitor and dominates geometric terminology (tangent, circuncírculo, etc.).
A lesson could be learned from the problems 1 (of the IMO 1969) and 2 - and usable for teens interested in math competition - is "if you can solve at home is better." At home, ie during pre-contest preparation.
Official Solutions
As in the textbooks that the solution of a problem is too compressed to see the official solution to the problems of a mathematics olympiad examination is often frustrating for the adolescent and their coaches, because it very often, enigmatic -To say the least. Is no exception for solutions to problems brought NOM jurors prepared.
then present them and remind readers that, in both math textbooks and in the issues of competition, you always need an intellectual
additional work to fully understand the solutions.
Solution to Problem 1.
n ^ 4 + 4r ^ 4 = (n ^ 2 + 2RN + 2r ^ 2) (n ^ 2 - 2RN + 2r ^ 2). Clearly the first factor is greater than 1, the second factor is also greater than 1 for r greater than 1. So we can take m = 4r ^ 2.
Solution to Problem 2.
Consider without loss of generality that the ray is inside the angle BAC. Then angMCA + angCAM angCMP = = = angCAD angMAD + angCAM. Moreover, angCPM = angCAD by subtend the same arc. Then, as the triangles ACD and MCP are similar, we have MC / AC = MP / AD. In addition, angACM = angNAD and angCAM = angADN. Therefore, the ACM and DAN triangles are similar. So AN / AD = MC / AC, and hence MP = AN and AM = NP as intended.

Solution to Problem 3.
couples looking all points (Pj, Pk), with 0 less than k jmenor below 21, that meet the segment that joins them is diameter. O equivalently, the length of the arc that joins them is 105. But as the length of the arc that goes from Pj to Pk is
j + (j +1) +...+ (k-1) = (k-1) k / 2 - (j-1) j / 2 ,
we should look for partners who meet k-1) k / 2 - (j-1) j / 2 = 105.
As (k-1) k / 2 - 105 should be positive integer or zero, and k = 15 is zero, then (P1, P15) is one of the couples wanted. The rest are giving ak value greater than 15 and verify if the result can be achieved with some j (j-1) j / 2. The partners sought are (P1, P15), (P6, P16) and (P12, P19).
Alternative Solutions and / or Constructive Feedback
constructive solution to problem 1
Note first that you want to factor n ^ 4 + m. That is, we n ^ 4 + m = (?)(?). The easiest thing is to complete the perfect square trinomial, and in the process, trying to guess how m. First, notice that we should make m = a ^ 2. So we have to do is complete the square in n ^ 4 + a ^ 2. Let's see:
n ^ 4 + a ^ 2 = n ^ 4 + 2 (n ^ 2) a + a ^ 2 - 2 (n ^ 2) a = (n ^ 2 + a) ^ 2 - 2 (n ^ 2) a.
However, for this expression is factorable, it is easiest to see as the difference of squares. Thus, a simple 2 (n ^ 2) a = r ^ 2.
But ... No. No. Here I have one 2 ... So I can improve my building by 2 (n ^ 2) a = (2r) ^ 2. But ... No. No. Not that either. The n ^ 2's I can leave out because it is a square. So it is better to 2a = (2r) ^ 2. Therefore, 2a = 4r ^ 2 or ^ 2 = 2r. So a ^ 2 = 4r ^ 4.
(Note that taking an initial idea as a perfect square m in general, it was a perfect square shape generated more specific the obligations of the solution is sought.)
After phase search and exploration of earlier (the discovery phase) and can be passed to the justification phase in the solution shown official. It would, leaving few traces of the search phase, something like
n ^ 4 + 4r ^ 4 = (n ^ 2) ^ 2 + (2r ^ 2) ^ 2 + 4n ^ 2 (r ^ 2 ) - 4n ^ 2 (r ^ 2) = (n ^ 2 + 2r ^ 2) ^ 2 - (2NR) ^ 2.
reader is left to the pleasure of completing the argument.
constructive solution to problem 2
The search for a solution to problem 2 below shows that is instructive of how to proceed in troubleshooting contest proves to be very effective in breaking the enigma that is the question. The statement is: Sean
c1 and c2 two circles intersecting at two points A and B. The tangent to by A intersects c2 c1 in C and the tangent to c1 and c2 A intersects D. A ray passing through A, inside the angle CAD, in M \u200b\u200bintersects c1 to c2 in N and the triangle ACD circuncírculo P. Prove that AM = NP.
part of the request. Ask you to show AM = NP. After a mental search for possible general strategies, one can conclude that similar triangles should be used. The strategy of similarity is almost infallible in such cases where there is a geometric configuration with overlapping circles. So like ... But what triangles ... Many! What we will do next is to find the right triangles, ie, pairs of similar triangles that would lead to the solution.
first thing you have to do is a good figure. The visual aid is valuable. Secondly we explore the possibilities of triangles to each side of the equation:
AM AMC's side of the triangle (note that triangle is the only configuration that AM is right). Now we focus on AMC triangle sides looking to link with others in the configuration: MC side of the triangle is MCP, and AC is the side of the triangle ACD (and ACP).
Now see the other side of the equation AM = NP. Note that here, as before, there is only one possibility - which makes us task. Now, as before, we focus on NPD to find the triangle linking with other triangles sides of the setup: DN DNA side of the triangle, and PD is next to the PDC.
To facilitate finding the appropriate pairs of triangles we form a crosstab: Side Triangle
AM AMC
AC MC MCP
ACD
NP NPD
DN DNA
PD PDC
This table and the figure should reach (after a while of searching) a pair of triangles ACD, NPD and AMC, PDC. For the purpose of developing a guess that we should look similar enough in Fig. Because there are other similarities that might link the two sides of the equality we want to reach, it is worthwhile to explore whether pairs and found lead us to something solid.
If NPD were similar to MCP, we would have NP / MC = PD / CP (note that we seek to NP and MC are present in the ratio). And if AMC would like DPC, would have AM / DP = MC / PC (note that we now seek to flirt as much as possible with the above.)
So, if you give these similarities, we would have NP / MC = PD / CP = AM / MC and arrive at NP = AM, as intended. So now we have to do is demonstrate that these similarities actually exist and we will finish. Left the reader the task. Only give the key suggestion to observe that the tangents from A to their respective circles are the key to see the similarities of angles required to establish the similarities.
Alternative method for guessing the similarities appropriate for problem 2
new parties of the request to show AM = NP, but now we will be forming ratios and proportions that do not affect equality, but at the same time be ligand triangles in the configuration:
AM = NP
AM / MC = NP / MC (the first reason and is, necessary to resolve the second)
AM / MC = NP / MC = (NP / PA) / (MC / CP) (the denominator and is, missing numerous)
(NP / CP) = (NP / PS) / (CP / PD) (and you are also numerous)
In summary, we have AM = NP is equivalent, in an algebraic sense, the AM / MC = [(NP / PS) / (CP / PD)] / (MC / CP) . And now look, visually aided by the figure, which corresponds to right reason on the left, again in a visual relationship of similarity. It is easy to see that AMC is like DPC and what remains is (NP / PS) / (MC / CP), which must be unity, ie, should be met similarity between triangles NPD and MCP. EUREKA!
Note: Again we reach the same conjecture, and, again, need to prove that the similarities do exist. There must also say that both procedures are heuristic, in the sense that it ensures that reaches a solid guess. And
also be emphasized that similarities in this search could continue to dead ends, before this thing to do is retrace the steps already taken and find another route. Conclusion
We wanted here to account for the Northeast VI Olympiad held in Cd Victoria Tamaulipas facilities UAMCEH-UAT on Friday September 22, 2006. The event was the importance added it was a symbolic act that inaugurated an era of government support for school mathematics in Tamaulipas. Additionally, it has been a pleasure to comment on the problems and their solutions, as well as a method of guessing similarities in geometry problems and useful for competition issues, allowing parties to focus gerométrica settings appropriate for the solution of the problem and avoid the teen "throw in the towel prematurely before the chaos of lines and points he has before his eyes could not decide on a promising path to solution.



 Elemental
Elemental