These open days at the University (of Tamaulipas) Saturday's workshop entitled "Science Workshop for young people." Responded to the call two high school teachers with 7 of its students, and a retired teacher.
The writer was in charge of the session with the intention to start developing the theme of "complex numbers and Euclidean geometry, a topic that I find very productive for solving geometric problems from an algebraic point of view.
The age of the participants (12 to 15) made me wonder, and better I ask you bring a problem of geometry that would like to address here with me? And his answer made me suspend the issue of complex and enter the matching of triangles, a common theme but has more potential than you might think to solve problems. Boys took out his notebook and I raised the
Problem 1:
In triangle ABC, with right angle at B, E and F are AC so that AB and AE = CF = CB. How long is the angle EBF?
Solution:
I decided to accept the challenge of solving (help) this problem is elementary geometry, however, their fine detail. I started with a discussion about drawing the figure and evoke theoretical meaning from the data.

The condition of equal segments seems to suggest using congruence of triangles. But once you see a figure closer (about especially after drawing BF and BE) the hypothesis of congruence should be replaced by isosceles triangles.
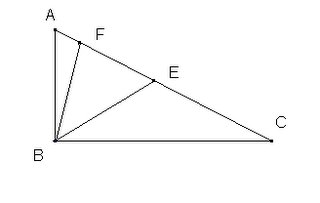
It is therefore clear that the triangles ABE and BCF are isosceles. And once you are bringing to mind the concept of an isosceles triangle, with it comes the "base angles equal."
So far, the cognizable is the expectation that the idea of \u200b\u200bequal angles at the base will be of some use. And yes. Because it allows the implementation of the algebraic machinery: M = x + y, N = y + z ... And an elementary teoremita was not mentioned (the sum of angles of a triangle is 180 ...) comes to save the whole situation: M + N + y = 180.

Since, moreover, by data we know that x + y + z = 90 ... a bit of algebra leads us to the answer y = 45.
us comment, finally, it is extremely rewarding experience for a math teacher to have a teen audience interested. It is indeed an extraordinary experience because it is common to have a captive audience (and the worst is that the teacher is also captive) with all the implications it may have the adjective. And one of them is the indifference of the majority.
While it is true that everyday classroom tend to negotiations for peaceful coexistence teacher-student, it is also true that most of the time these covenants courtiers are not entirely satisfactory to the parties - at least for the teacher who is trying to satisfy two conflicting forces: the duty to be of quality education in response to a society that naively still waiting for the educational system and the educational reality has used students to make paper without any effort on your part. Neither good nor bad, it's just a fact of life in Mexico. (Does the fact that the OECD we stand at last in the long run might change the situation?)
JMD in VL greets ... and promises to post more often ... at least one problem was solved in the Saturday session of the workshop ...
0 comments:
Post a Comment