IMO, Mérida, solving problems as a sport
In July 2005 international mathematical community heard about Mexico (and the Mayan culture) through the city of Mérida hosted the International Mathematics Olympiad, maximum to which they aspire to reach teens who have decided to study mathematics from a sporting point of view.

Merida (and the Yucatan peninsula) evoke "natural" (at least here in Mexico) Maya culture, and in particular, Chichen-Itza (and the pyramid of Kukulkan) - a of impossible to ignore the traces of a glorious past, a vanished culture. (The photo is of José Muñoz Porras, who happens to accompany the delegation of Taiwan during the week of competition.)


But let me comment on the problem 5 of the competition, an issue that touched Jesus Rodriguez Viorato review and who promised to work that problem before he himself said in a talk by Congress of the Mexican Mathematical week from 24 to 28 October 2005.

The problem
Let ABCD be a convex quadrilateral with sides BC and AD equal and not parallel. E and F are points on sides BC and AD respectively such that BE = DF. The lines AC and BD intersect P, the lines DB and EF intersect at Q, the lines EF and AC intersect at R. Consider all triangles PRQ as E and F vary. Circuncírculos demonstrate that these triangles have another common point other than P.

Comments exploratory
The first thing you have to do in competition issues is an act of faith: what is being asked to prove is true. In this case, if requested to demonstrate that there is another common point to the PQR circuncírculos is that it exists. And the first task is to guess what that point is, tentatively characterize it then goes on to show that this is indeed the other point.
A good figure (well designed) can help. In fact, active exploration is needed on the initial figure. A good attitude for the solution of problems of competition is to reason as follows: if there is another point (and there must be since it is asking for your show) then that point must be one of those belonging to the configuration of the initial figure or likely to be characterized well in it.
For example, a point of intersection of two geometric objects can be generated from the figure. (It's like saying "! Looking for a mathematician!" And one that has to do is look at where the mathematicians tend to be - For example, at the Institute of Mathematics. I mean the places where we can find with probability one.)
EF
With two segments can locate the second point, call it T, circuncírculos for drawing the triangles PQR. But it remains to be seen how the objects is characterized by the figure (or other auxiliary constructed from those).
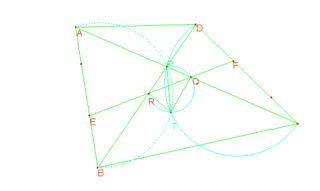
However, once located T graphically, one can somehow use the fact that (in a translation of the request) PRTQ configuration is cyclic quadrilateral (ie, if T The other point was then ... if we are to believe the veracity of the request). And that leads us to draw lines cyclic quadrilateral characteristics - such diagonals.
And what you want is to place T with respect to known geometric objects (in the settings given in the statement), not yet finished. You need more exploration. With a little luck (and perhaps experience similar problems - that I have and so I took much more time) can have the idea - a little more experimenting with the settings, perhaps because of the way it is T located in the figure - nothing to lose circuncírculos trace the APB and CPD, and it is there where does the discovery: T is the other point of intersection of these circuncírculos. (And the best part is that we can draw the whole theory of the geometry of the circle! - And in particular the cyclic quadrilateral)
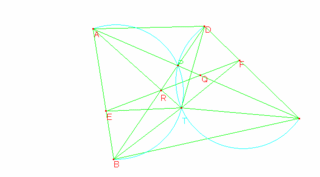
targeting only certain key ideas of the show to avoid overloading this speech in text mode so geometric notation:
1) We need to find the points of interface between what we know (or can easily tell from the figure - with already Helper Objects -) and what we want knowledge, ie, show that PQTR is cyclical. An example: the angle is cool TPQ of TPA, but TPA is one of the angles of cyclic quadrilateral ABTP, the angle TPQ is thus a good interface.
2) It is easy to get lost with so many associations, and subsequent discoveries, angles (and their consequences), for example, in the figure are three isosceles triangles, but only two are used. So the compass should be the goal that you want to go, in this case the goal is to demonstrate PQRT cyclical (and shown from two angles to see that characteristic are equal). And if you have an angle (the QPT) then you must target the appropriate (the TRQ). Thus, the TRQ to the angle should be the compass.
3) The time immediately before reaching the angle TRQ is to discover that EBRT is cyclic quadrilateral, to which you get from the discovery that the isosceles BTD and ETF are similar. (This is done to see that the angles EBT - we already know from the beginning that is equal to the TPQ - and TRQ are supplementary angle of the ERT, and therefore are equal.
didactic digression (and design instruction in geometry)
From a teaching standpoint, the problem 5 of the IMO 2005 (and probably all the other 5, but those not yet explored) is already a repertoire of skills and knowledge in geometry that translate easily leave a training program.
And find one from the statement. Check out this: "Show that circuncírculos ..." The very term "circuncírculo" reflects primarily a teaching sequence: definition and understanding, from many years of construction, for example, the method of construction of a circuncírculo, its properties, the same for incírculos, excírculos, etc. And from there, the method of construction would lead to a dip straight from the triangle (medianas. bisectors, angle bisectors, etc..) And its associated theorems ...
Also in the statement, the phrase "as EF varies" (which should be understood "varies maintaining their properties") points to a training set reading skills - especially in reading "between the lines" of statements. And in recognition of the fact that, in a statement (and any speech), not everything is said, that certain holes should be filled by the reader.
On the discovery (the other point), guess what's the point T is a challenge - though perhaps not for many young people in Merida solved the problem in about two hours. The discovery phase aims at training forms and methods of exploration and experimentation with geometric configurations. Especially since a dynamic point of view (as EF varies). Particularly aimed at training in the use of geometry software (CBBRI, GSP, Cinderella, ...).
The way I discovered the other point may be illustrative. I used the CABRI (the French equivalent of Geometer's Sketch Pad). First drew the settings according to the statement and then circuncírculo for PQR. This did vary EF (as easy as moving the mouse one end). And I watched how he moved the circuncírculo.
But that did not help much to find the other point. So I drew on the settings you already had another line EF to have two circuncírculos and see what was the point remained fixed. Again moved with the mouse one end of a line EF and observed the dynamic behavior of the geometric configuration. So I saw that there was indeed another point (apart from P) that remained fixed during the movement of EF. But no more.
The check that there was another point he gave me some confidence (he had a psychological effect) but there was still characterize the fixed point T in terms of configuration objects. And perhaps it was the way T was located in the figure, with respect to other objects, which led me to more experimentation - all case, the geometry software, the experience costs almost nothing.
Another idea that helped me achieve and I guess before but I repeat it here: confidence in the axiom (axiom might be called geometric problem solving Olympiad) that T should be a starting point of the buildable and objects in the configuration.
It also has a psychological effect of discharge of anxiety, but also very real, and perhaps there could be a research project on this kind of statement, apparently mysterious but in which the clues are there in the words of those statements - the word circuncírculo I hovered in the head ...
So I built (with the CABRI) the PBL and CPD circuncírculos y. .. Oh revelation! Indeed, there was clearly that the point T is the other intersection of these circuncírculos.
In summary, I would like to stress here that the use of geometry software maximizes the efficiency in solving geometric problems. But above all the learning efficiency in solving geometric problems - since the contest only allowed the case of geometry.
Incidentally, and as a recognition astonished the young contestants, which is a feat of those boys - from those that solve the problem IMO 5 in 2005 - to have guessed the point T with ruler and compass (and have shown that this was indeed) in the short space of two or three hours - as they have 4 and half to solve the three problems. (Do not rule out the possibility that experienced similar problems, some have started the problem with "I'll show you the other intersection point is the other ..." and have saved the search and examination process to achieve conjecture.
Finally, let alone the end of these comments, the teaching or lesson that leaves the solution of a really complex problem, as is the problem 5 of the IMO 2005, is an experience life (or almost): the memory remains long in our minds. As I recall, that is, the configuration of relations between its objects, the demonstration plan, the intermediate steps ...
and clarified that what I present here is a reconstruction of a search that took me two days (and a third to draw up the reconstruction). And perhaps someone could say "what a waste of time!". And I would say "well ... everyone loses according to their own aesthetic preferences and trends ..." (What a pain! I'll have to catch up on the private lives of famous TELEVISA what antics have made REBEL those?! Gossip Pásenme please!)
Sincerely JMD in VL

