On the notion of congruence of triangles
Equality and consistency
The concept of congruence is related to the equal and it is expected that the learner knows it, either intuitive meaning from natural language or through use in arithmetic. It is customary to speak of congruence geometry rather than equality. For example, two segments are congruent if and only if they have the same measure, and the same is true for angles. But in the case of two triangles, the definition is more complicated because there is no measure (number) that defines a triangle.
triangle as a configuration of points and lines
As we know, there are different classifications of triangles that account for their diversity of form: according to the measure of their angles can be obtuse, rectangles, acutangula, in accordance the relationship of the measures of its sides can be equilateral, isosceles, scalene. That's why a pre-defined notion of congruence of triangles is the correspondence. This is because a triangle (and any polygon) is a configuration consisting of points and line segments (sides) that connect pairs of points.
Congruence triangles as intuitive notion and its formalization
Having discovered that two triangles are congruent (equal) should put their corresponding vertices. To say that the triangle ABC is in correspondence with IJK means that the correspondence between its vertices is AI, BJ and CK. And in this correspondence is implicit in the correspondence between the sides: AB-IJ, JK and BC-CA-KI. But it is also implicit correspondence between the angles: the angle at A is congruent to angle R, etc. (Note: not all texts follow this convention, that is, even when claiming "ABC is in correspondence with IJK "do not respect the above rules of implied correlation-a shame ... but what are you going to do.)
And when I say" discovered "I mean the view consistency cognizable by intuitive and informal methods, or perhaps rather, "sees." But once you "see" the consistency should be formalized. This is desirable because once the correspondence and consistency in the way explained above, it is not necessary to see the figure to raise equations or reasons, then the correspondence between vertices and sides are implicit in the correspondence between the triangles as already explained.
To see the need to search for consistency, that is, something (a sentence, a fact, ...) in the problem statement to suggest that consistency can be used for its solution. And to find it, once you seek it is convenient to use the intuitive definition: two triangles are congruent if they can be matched one on the other by rotations, translations and / or reflections. (The formal definition is: two triangles are congruent if, in the correspondence between their vertices, are equal to the corresponding sides and corresponding angles.) In a triangle congruence then have six pars, three sides and three angles. It is therefore very useful have criteria that tell us whether two triangles are congruent without having to verify the six equalities.
matching criteria as postulates
The criterion (principle) of consistency is perhaps the most basic criterion called LAL (side-angle-side) tells us that if, in a letter of triangles, two sides of one and the angle between them are equal to their corresponding elements in the other, then the two triangles are congruent. Some texts of formal geometry, the most in the logical sense, taking this approach as an axiom and show the remaining two, the ALA and the LLL. Other texts-most- postulated as true the three criteria. It is recommended then that the learner's take the three as postulates for if in any way is going to take a postulate ...
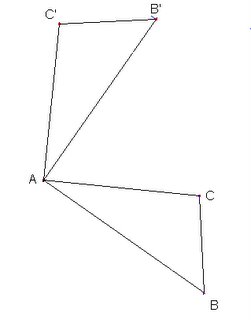
In the figure, the triangles ABC and AB'C 'are in correspondence. The second is the result of the first rotated 90 degrees. If the missing segment BC, however the distance between A and B would remain after the turn.
Instance of use (classical) of the LAL test
isosceles triangle theorem:
If a triangle is isosceles then its base angles are equal. (Note: it is customary to understand the basis, the third side, the first two are the ones who know the same.)
Demo:
Warning: This instance of use is somewhat disconcerting when you first see it, so it asks reader's cognitive cooperation. (The confusion is perhaps due to the triangle is placed in correspondence with himself, which is not forbidden but because one thinks that this ban was implicit in the definition of consistency.)

The isosceles is sample can be called triangle ABC. But, crossing the vertices in the opposite direction can be called triangle BAC. Correspondence is valid for ABC-BAC.
Since the triangle is isosceles with CA = CB and BC = AC. Also, since it's the same triangle, the angle at C is identical to itself. There is therefore a correspondence LAL and the two triangles are congruent. But then the other elements put in correspondence are also equal. In particular the angle at A is equal to angle B.
second instance of use (also classic) the LAL test
In an isosceles triangle, the bisector of the vertex opposite the base divides the triangle into two congruent.
Demo:
In the above figure draw the bisector of angle C and assume that intersects the side AB at M. By hypothesis and MCB ACM angles are equal. This suggests the CC correspondence. On the other hand, by definition, AC = CB. This correspondence suggests AB, and the other point common to the triangles formed by the bisector is M, which suggests the MM correspondence.
Thus, we test the correspondence ACM-BCM. We have, AC = BC and CM = CM, to be seen whether the angle formed by AC and BC is equal to BC and consisting of CM. But that is true because CM bisector. So we can use the LAL test to establish that the positions corresponding triangles are congruent. This congruence
well established are still several
Corollaries (for isosceles):
a) The bisector is also bisector (as AMC and BMC angles are equal and their sum is a plain, but also the corresponding sides AM and BM are equal, so that MC is perpendicular to the midpoint of the base)
b) The bisector is also medium (for AM = BM)
c) The bisector is also high (as AMC and angles BMC are straight) Final comments
can deduct the standard LAL LLL from applying the properties of an isosceles triangle, the triangles LAL is placed in correspondence as shown in the figure and ...

Since AB and AB = IJ = IK, we have the isosceles ABI and ACI. But then its base angles are equal. Adding, we find that the angles at A and R are equal and we are now able to apply the LAL test to ensure that the triangles ABC and IJK are congruent.
Say, finally, that the notion of congruence of triangles is very close to the foundations of Euclidean geometry. But the apprentice does not need to justify everything, especially near the foundation theorems. It is better, from the point of view of solving problems, to take the matching criteria as axioms and shamelessly use in solving problems. This allows you to move forward in its appropriation of theoretical tools without wasting time on formalities. Also be taken as equal angles formed by two parallel and a transversal. Of course it is desirable that some may see demonstrations of the basic theorems, but that can wait ... Meanwhile, to solve problems ... in VL
JMD
greets